At an increase of temperature, a molecule of gas receives energy, becomes more mobile and capable to borrowing greater volume. And if the volume is limited, the pressure is increasing. The increase of temperature leads to destruction of intermolecular connections in the gas, and the gas passes into an atomic state. At the further increase of temperature, the connections between the kernel the electrons in electronic environments fall. First, the electrons from external environments are lost, and the more energy an atom receives, the more close to the kernel electrons leave the levels. During this period, an electronic gas appears, atoms become ions — the gas passes into the state of plasma. In this state, due to the occurrence of additional electronic gas which, represents highly mobile electrons, the volume of the plasma increases strongly, and due to the restriction of volume the pressure increases. At the further increase of temperature, all the electrons are leaving the atoms and kernels of atoms appear in the gas-plasma mix. Thus, the gas-plasma mix can be consisted of gas molecules, gas atoms of, ions of atoms, electrons (electronic gas) and kernels of atoms. The weight of the gas-plasma mix consisted of:

                   M_GP=M_g+ M_A+ M_Io+ M_e+ M_R + M_GL + M_O                                                       (5.3)

Where M_g — gas weight in the gas-plasma mix;

             M_A — atoms weight in the gas-plasma mix;

             M_Io — ions weight in the gas-plasma mix;

             M_e — electronic gas weight in the gas-plasma mix;

             M_R — free kernels weight in the gas-plasma mix;

             M_GL — the weight of the substance transferring from liquid and firm states into a gaseous state and plasma, and back from plasma and gas into liquid and firm state, due to the change of pressure and temperature;

            M_O — the weight of neutral and unconsidered particles.

               Weight of a star:

                             M_Z = M_GP + M_BK + m_Iz                                                                        (5.4)

Where M_BK — the weight of «the white dwarf» — a firm kernel of a star;

             m_Iz — the weight of star radiation in a unit of time.

In order to sort out the equation of the gas-plasma mix state, it is necessary to make the equation of the state for each addendum in the formula (5.3) which can be written down in shorter form: 

                                

             M_j — the weight of j component of the formula (5.3).

While forming the equation it is necessary to consider the influence of magnetic fields of the star on the action of the gas-plasma mix.

                               

Where Φ — parameter of the magnetic fields;

   Δp_Mg — pressure change in the gas-plasma mix, under influence of the magnetic fields of the star;

   v_GP — the volume of the gas-plasma mix;

   f_Φ(Φ; M_GP) — functional dependence on the parameters of magnetic fields of the star and the weights of gas-plasma mix M_GP.                                                                                                             

  The equation of the state for the gas-plasma mix will have dependence on the weight of the mix, on the temperature, on the chemical compound and on the influence of magnetic and other fields.                                                                                               

                             p_GP ^. v_GP =f(M_GP; T; Φ; x)                                                                   (5.5)

  f(M_GP; T; Φ; x) — functional dependence on the parameters of the gas-plasma mix of a star.                          

The pressure of the gas-plasma mix can be defined by the following formula:

                                                                                   (5.6)  

Or                           

For addendums p_e and p_Mg the formula (5.9) is not true. Since p_e – is proportional to the pressure of electronic gas which, consisted only of electrons, and p_Mg can be considered as an influence of the magnetic field on all of the gas-plasma mix. But if to consider p_Mg as the sum of changes of every addendum p_j, under the action of the magnetic field, and for a case of p_e to accept that i=1 then, for p_e and p_Mg the equation (5.9) will be fair. Hence, the formula (5.3) can be written as follow:

Apparently from the given analysis, the conclusion of the equation of the gas-plasma mix state is inconvenient these days and in the future we shall use the formulas:

                                         p_GP ^. v_GP =f(M_GP; T; Φ; x)                                                            (5.5)

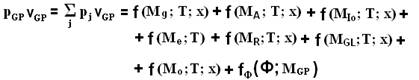                        (5.8)                        

                                                                                                 (5.6)

                                                                                                    (5.10)

                                                                                                        (5.7)

For defying the force acting on the expansion of a star, it is necessary to multiply the pressure of a gas-plasma mix by the surface area of the star.        

Where S_Z=4 ^. π^. (R_Z)² — surface area of the star;

             R_Z – radius of the star.                                  

                            

R_BK – radius of the star’s firm kernel — «the white dwarf».

At the end of life, the gas-plasma mix gets warm; aspiring to increase its volume, the density of the «fuel» is lower than at the beginning of a life. The thermonuclear synthesis is fading, the force of compression decreases, and there are fewer forces directed on the expansion of the gas-plasma mix. The star transfers into the state of «the red giant», the mix extends, and the pressure decreases. Part of the liquid and the firm substance of the star transfer into a gaseous state, increasing the volume and the weight of the gas-plasma mix and of «the red giant». The heated gas-plasma mix of the star supersedes the cold space dust and gas from its volume. Considering that the star wind supported the unloaded space around of a star, the expansion of hot gas-plasma mix will not meet strong resistance from gas and dust of space among the stars. Regarding the gas streams in space, easing or disappearance of the star wind create a vacuum area, in the volume of a hemisphere of a star where, the cold gas stream from a space is soaked and rushes. Further there is a process of cooling of the star’s gas-plasma mix of during which the plasma transfers into a gas state. Kernels of atoms, ions and electrons lose energy and speed, and this allows the kernels and the ions to grasp free electrons. The electronic gas, taking most of the volume is absorbed; the volume of the gas-plasma mix is quickly decreasing.                             

Simultaneously there is a decrease in the mix volume, due to the cooling. It leads to a very fast reduction of the gas-plasma mix volume. The fast decrease of the mix volume leads to the occurrence of an additional vacuum, relatively to a very low pressure of the interstellar gas. The vacuum formed around the kernel of the former star soaks up the dust and the gas from space. If the parameters of the star and the gas of the space around are matching the values necessary for the synthesis renewal, hence the dynamic process of absorbing gas from space is transferring to the process of atmosphere compression in the «the white dwarf», with the subsequent renewal of thermonuclear synthesis in the atmosphere of «the white dwarf». This process «lights up» the star repeatedly. If there is second star around, than at the appropriate characteristics of both of the stars, the dying star sucks  the gas-plasma mix of the star that is still burning along with the gas stream and dust of the cosmic space around them.

 In the crown of the chromospheres and photosphere second star, a «hole» is created because part of the substance is soaked up by the vacuum around «the white dwarf», and through this «hole» the gas-plasma mix tries to extend and is being grasped by the stream which moves to the «dying» star.
The fact of secondary «ignition» of a star confirms the hypothesis that synthesis in stars doesn’t occurs in the kernel, but in the atmosphere.
This kind of chain of physical events helps to explain the repeated «ignition» of a star around the white dwarf.
And so, we have received the «virtual» for meanwhile equation of the gas-plasma mix state. In the received equation, the parameter M_j acts relatively the same as the temperature T, since mass values of the components of M_j are determining the characteristics of the gas-plasma mix of the star.                       
More competent researchers should do a more exact conclusion of the equation of the gas-plasma mix state.                

The gas-plasma mix plays one more important role in the star’s design. The gas-plasma mix is the shock-absorber of the dynamic processes in the star’s atmosphere. Hence, as a result of the dynamic processes in the top layers of the star on the gas-plasma operates a load which has dynamic character. This dynamic load, aspires to forms to a distribution on the whole volume of the gas-plasma mix and through it to the surface of «the white dwarf» — the kernel of the star, is similar to «an air pillow» and to the process of distribution of load in the air chamber of a wheel of a car.                                                                                            

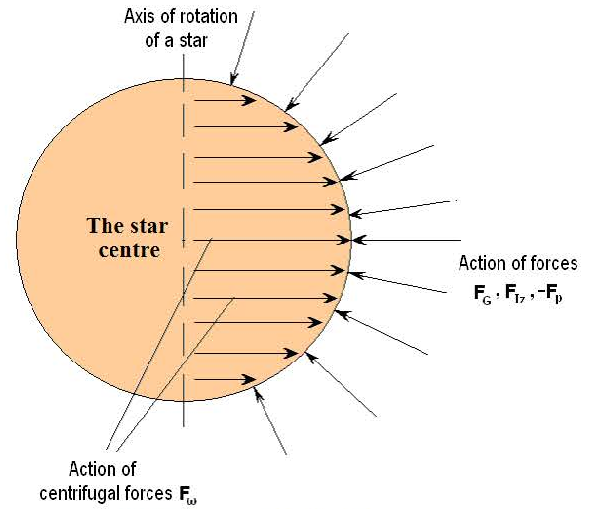
  2. The force operating on the gas-plasma mix as a result of the start’s rotation or centrifugal force F_ω.

At first sight, the conclusion of the action formula of the centrifugal forces on the gas-plasma mix isn’t difficult, and it is also similar to the conclusion of the planets formula. But a detailed consideration reveals that the task is not that simple. In a general view, the centrifugal force is defined by the formula: 

Where ω_i — angular speed of rotation in i point;

  l_i – the distance, from the point we’re interested in, up to the rotation axis.

Let’s disassemble an example of the Sun for figuring out the complexity of calculating the centrifugal force consists in a star. Action directions that we considered earlier were processed through the center of a star. That means, the action of these forces is presented in all planes and directed to the center of the star from different directions or from the center of the star to all of the directions. The Centrifugal force operates in two planes, perpendicular to the rotation axis and along all the rotation axis of in the stars aisles. And only on the equator, where the centrifugal force is maximal, the direction of its action passes through the center of the star (figure № R-5.1). 

                                                                      (85)  Рисунок № R-5.1

The second difficulty of calculating the action of centrifugal forces is the non-uniformity of the gas-plasma mix movement.