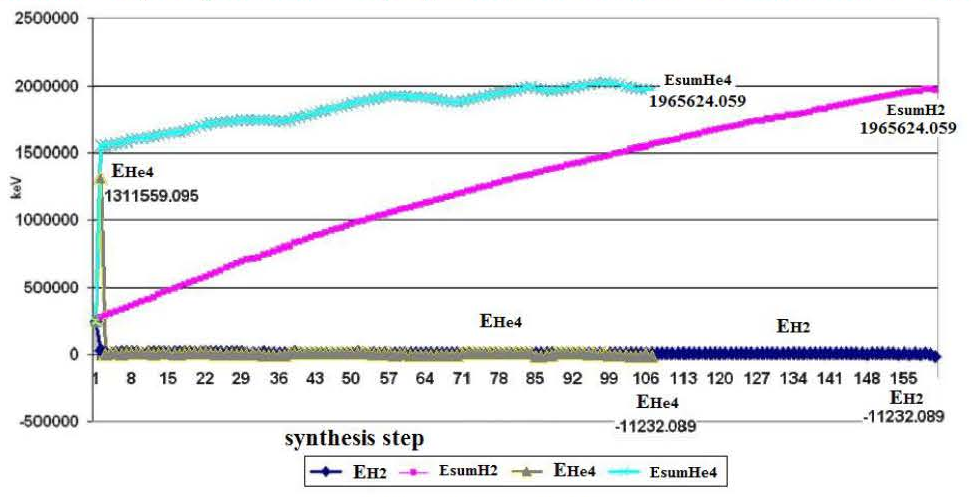
Allocation of energy at synthesis of kernels, under the schemes:

                   «A kernel + Hydrogen with A=2 (H2)» and «the Kernel + Helium with A=4 (He4)».

                                                                           (41)  Schedule №G-3.6

From schedules №№ G-3.5, G-3.6 it is visible, that in the considered case, most of energy is allocated in the field of the synthesis of easy kernels (H-2 and He-4). During a further synthesis of a kernel, the allocation of energy is much less.                                                                                                                         

Hence, and in this case there is a compression of the central areas of a star that confirms the received earlier results and the conclusions drawn by us.

Forces of compression not only condense the matter of star, but also keep its gas atmosphere.

 Let’s analyze variants when in a synthesis not one, but two, three or ten easy kernels are being participated. That means, that in the synthesis the participants are the initial kernel and two, three, or ten easy kernels of hydrogen H-2 or helium He-4. We shall make the calculations of the allocated energy at the synthesis by the formula:

                              E_c.k = (M_a + k ^. M_b — M_c) ^. C²                                                        (3.18)

Where E_c.k — the energy allocated at the synthesis of a kernel c;

M_a, M_b, M_c — weights of kernels a, b and c;

C — speed of light;

k — quantity of easy kernels participating in one act of synthesis of a kernel c,

     (k=1; 2; 3; 10).

Kernel a — the initial kernel participating in the synthesis;

Kernel b — easy kernel of hydrogen ₂¹H or helium ₄²He;

Kernel c — kernel received as a result of synthesis of kernels a and b.

But, since it is more convenient for us to use parameter ΔD from

№ A-1 in calculations, the formula (3.18) will look:

                            E_c.k = ΔD_a+ k ^. ΔD_b – ΔD_c                                                     (3.19)

The chains of synthesis on which allocation energies are calculated are presented by reactions in the table № T-3.6.

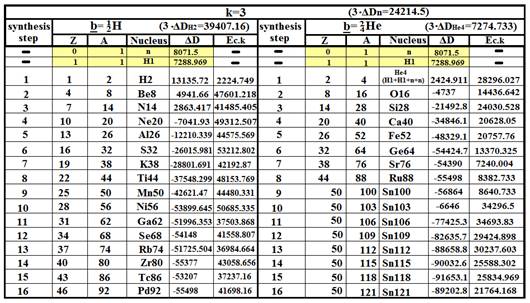
                                               (42) Table № T-3.6. Part A, (k=2)

           Chains of synthesis and allocated energy by reactions, participated in the

                                                synthesis of several easy kernels. 

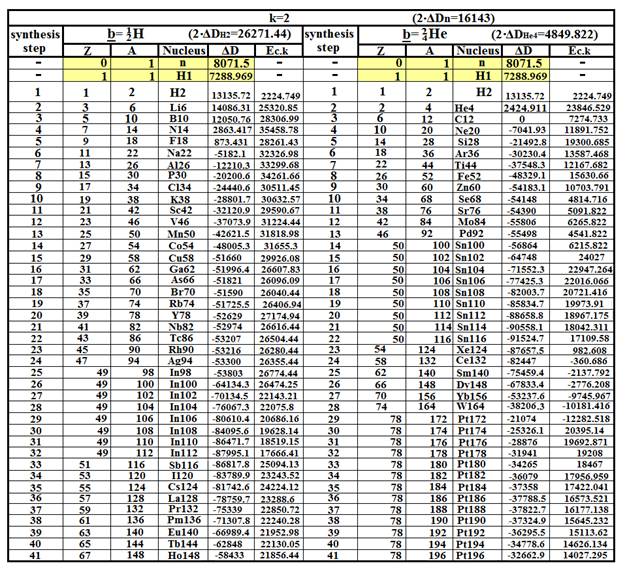
                                                            (43) TABLE № T-3.6, a part-A

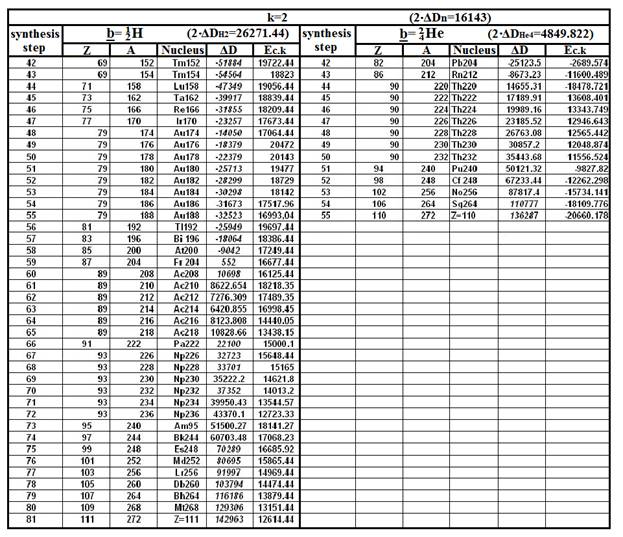
                                                            (44) Table № T-3.6, a part-B. (k=3)