2.5. Speed of kernels at synthesis

We know that for the synthesis of two kernels and more, it is necessary, that at least part of them will have kinetic energy and a speed sufficient for overcoming the coulomb barrier.

Where from a kinetic energy for synthesis appears in kernel?

Let’s consider the process we’re interested in on the example of three kernels: «A» and «B», which after the synthesis turning into a kernel «C». And this kernel «C» should participate in the following acts of synthesis.

Where from a kinetic energy for synthesis appears in kernel «C»?

It’s very simple, during the synthesis of kernels «A» and «B», their impulse is transferred to kernel «C», but at this act of synthesis, an energy in the form of radiation is allocated:

                                E=Δm ^. c²                                                                            (2.33)

                                Δm=m_a+m_b-m_c                                                                   (2.34)

   Where m_a — weight of kernel «A»,

         m_b — weight of kernel «B»,

         m_c — weight of kernel «C»,

         Δm — a difference between the sum of weights of kernels before the synthesis and the weight of the received kernel «C» after the synthesis (defect of weight),

        c — The speed of light.

The impulse from this radiation is being transferred to kernel «C», according to the third law of Newton.

Hence, the impulse of kernel «C» depends on the impulse of kernels «A» and «B» and on the impulse received during the radiation of energy Δm ^. c².

                 P_c= m_c ^. v_c = f(m_a ^. v_a; m_b ^. v_b; Δm ^. c²)                                           (2.35)

  Where P_c a-impulse of a kernel «C»,

        v_c-speed of kernel «C»,

        v_a-speed of kernel «A»,

        v_b-speed of kernel «B».

Let’s deduce the formula for the impulse of a kernel

                                   P_c= P_a +P_b +Δm ^. c                                                         (2.36)

  Where P_a; P_b; P_c — impulses of kernels «A», «B» and «C».

Full energy of any kernel is expressed by the formula:

                             W_j=E_j+m_j ^. c²                                                                        (2.37)

  Where E_j — kinetic energy of a kernel j,

              m_j — weight of a kernel j.

It is possible to present and an impulse of a kernel in the same way. For this purpose we shall substitute the formula(2.34)(Δm=m_a+m_b-m_c) in the formula (2.36)

                           P_c= P_a +P_b +Δmc = P_a +P_b + (m_a+m_b-m_c) ^. c

                          P_c+m_c ^. c = P_a + m_a ^. c + P_b +m_b ^. c                                        (2.38)

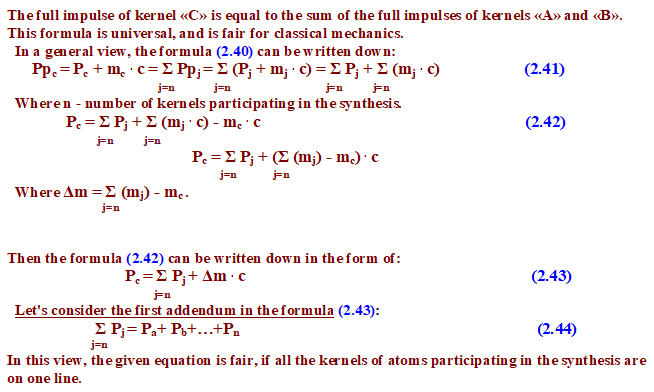
Let’s name Pp_j — a full impulse of a kernel:

                                       Pp_j= P_j + m_j ^. c                                                               (2.39)   

Full impulses of kernels «A», «B» and «C» will have the following appearance:

                                    Pp_a= P_a + m_a ^. c                                                                              

                                    Pp_b= P_b + m_b ^. c                           

                                    Pp_c= P_c + m_c ^. c     

Then, the formula (2.38) can be written down:

    Pp_c= Pp_a+ Pp_b= P_c + m_c ^. c = P_a + m_a ^. c + P_b + m_b ^. c                                (2.40)