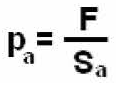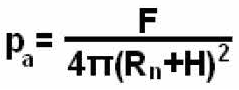On the surface with a point «A» under the action of force F, the pressure directed to the center is created:

                                                                                           (2.29)

  Where, S_a=4 ^. π ^. R_a² — the area of a surface of a sphere with radius R_a.

If will go lower to the sphere on depth «H», on the level of point «N», we shall appear on a surface of sphere, with radius R_n.

                                          R_n=­­ R_a – H

                                          R_a=­­ R_n + H

The area of the surface of the sphere with radius R_n can be calculated in the formula:

                                           S_n=4 ^. π^. R_n²

The area of the surface of the sphere with radius R_a:

                                    S_a=4 ^. π^. R_a²=4 ^. π^. (R_n + H) ²

         That means S_a> S_n

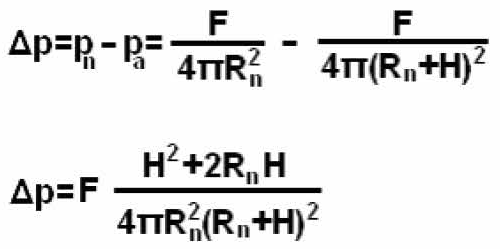
Difference between the areas ΔS = S_a — S_n        

            ΔS = 4 . π. (Rn + H) 2 — 4 . π. Rn2=4 . π. (2 . Rn . H + H2) 
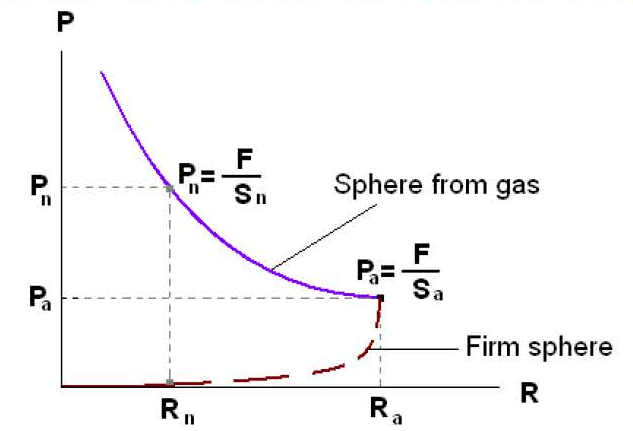
The force acting on areas S_a and S_n is the same and equal F. As, the area of a surface of a sphere with radius R_n is less, hence, the pressure in point «N», will be higher.

                                                                 (2.30)

Pressure in point «A» is equal:

                                                                  (2.31)

From formulas (2.30) and (2.31) it is visible, that at a decrease in the area of the surface of the sphere, the pressure upon this surface increases. The difference of pressure on different layers of the sphere can be defined in the formula:

                                   (2.32)

From the last formula it is visible, that at the decrease of R_n, the pressure acting on a layer with radius R_n increases, and Δp increases between layer «A» and layer «N».

From the stated above, the pressure created by force F and directed into the center will increase with the approach to the center of the sphere. In other words, in our case there is a concentration (accumulation) of force in the center.

This peculiarity, concentration of force, radiation, energy in the center, is one of the most important peculiarities of the spherical form. Probably, due to this peculiarity, there are processes of synthesis both easy and heavy kernels in stars.

   According to calculations of reduction of radius of the sphere, the pressure directed inside this sphere increases and reaches huge values. So on distance R_k =0.282 m from the center, and with area of the surface of the sphere equal to 1 m², value of pressure acting on this sphere, will be equal to value to all the force of compression on the surface of a star. At smaller radius R_k the pressure increases sharply. Whether such increase in pressure is probable and how the matter of a star behaves at such high pressure and a heat, remains unknown for us meanwhile. But we can predict it. We know that with an increase in pressure, the density of gas increases up to liquid and firm state, that possibly occurs inside of a star as well. Helio-seismological researches of the Sun speak about the existence of a firm kernel in the center of the star.

Let’s disassemble another peculiarity of the spherical form, but of a firm body, yet, it’s not less surprising. Under the same conditions which we have considered for the gas sphere, for a sphere from a firm matter, the distribution of loading will be absolutely opposite.

  That means, the loading acting on the surface of the sphere, from a firm matter, with approach to the center of the sphere, does not increase, as it is observed in a variant with gas, and on the contrary – it decreases, and reaches the minimal values in the center.

All basic loading is accepted with external layers of the firm sphere.

This peculiarity of a firm sphere is not studied in conditions of stars yet and requires serious researches.

Whether the existence of a firm body is possible at such heats and pressure, and what values of these parameters exist in stars, we do not know yet. According to helio-seismology it is possible to draw a conclusion, that a kernel of the Sun is firm or liquid, or a combination of firm and liquid matter.

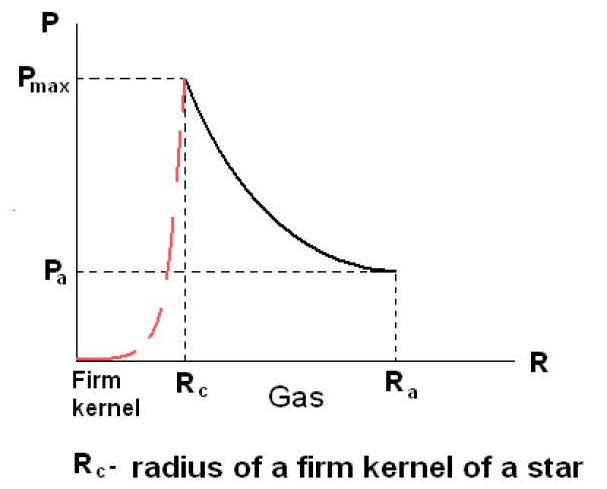
Let’s consider graphically, on the schedule №G-2.2, the distribution of loading in a sphere from gas and in a sphere from a firm matter. We do not consider action of gravities.  

                  (13)  Schedule №G-2.2

Consider the graphical load balancing in the star, taking into account the gaseous envelope and solid nucleus, provided the action on the surface of the star of the dynamic force F, directed towards the center. Load change under the influence of gravity, we do not consider.

                  (14)  Schedule №G-2.3

In schedules №G-2.2 and №G-2.3 we do not consider the change of pressure in a liquid sphere, though it is possible, that on border between gas and a firm kernel there is a matter in the liquid or fused state. It is probable that this liquid layer aspires to distribute in regular intervals loading on the surface of the firm kernel. Change of pressure in a liquid probably has character similar to the change of pressure in a gas sphere.

Apparently from the schedule №G-2.3, the pressure created by dynamic force in the top layers of a star, increases with the approach to the center of a star in square dependence, up to the maximal value, at the reach of a surface of a kernel. The liquidly-figurated layer of matter on the surface of a firm kernel of a star is aspiring to distribute the dynamic loading in regular intervals on the surface of the firm kernel.