Physics of the Sun and stars
3. The power analysis stars (Sun), as nuclear reactor
— Method of maintaining power analysis
In this case, the spontaneous (casual) method and the method of «technology of process» are applied. That means, the method of «technology of process» is applied casually (spontaneously).
In the given method, the main criterion is the sequence of events occurring during a process, or a process of consecutive events. Let’s say, we investigate process in space, in the environment, or inside of an object, on which a direct research is impossible, the process is hidden from us. In the given process, the element «A» turns into element «D».
There is a task in front of us, to predict, by using the modern knowledge, the possible technological chains of transformations of an element «A» into element «D», and to define by these chains the events and the processes which are occurring inside of the investigated phenomena, processes, or objects, and if possible, the structures of these objects. The existence of intermediate elements, «B» and «C», simplifies the forecasting of «technological chain» transformations of element «A» into element «D».
In a star, there is a process of transformation of hydrogen in heavier elements of the periodic table. Having predicted the possible variants of the given process of transformation, it is possible to define the events and the processes which are occurring inside of a star. Knowing, the events and the processes occurring inside in a star, considering the necessary conditions and design features, for the creation of these conditions, events and processes, it is possible to predict a structure of a star, as a reactor for synthesis of kernels.
Possible «chains» of synthesis of kernels are made theoretically, from hydrogen up to kernels of atoms with Z=111 and A=272, being in the end of the periodic table of elements. «Chains» of allocated energy are being made, and their analysis has led to a conclusion, About necessity of correcting of modern physics of stars and the Sun, and of the theories about their structure. We consider the device of a star, as the device of a nuclear reactor in which, technically and technologically there should be conditions for a synthesis of kernels of atoms.
— Physical bases of the power analysis
The star is a huge nuclear reactor in which, there are nuclear reactions. Is the existence of such huge energy source and storehouse of fuel simultaneously is possible without the law which copes this miracle?
Whether it is identical, the allocation of energy during the synthesis of kernels of atoms on all periodic table with mass numbers from 2 and more 200 (A> 200)?
Why during the allocation of huge energy the star does not break off?
The analysis of allocation of energy will respond to these and some other questions during the synthesis of kernels on a chain from a proton and a neutron up to the heaviest kernel with Z=111 and A=272.
In this chapter, we shall consider the laws of allocation of the energy, operating at moment of the synthesis of kernels of atoms in a star.
Defect of weight of a kernel is the difference between the actual weight of a kernel and the weight of all nuclides (protons and neutrons) of this kernel in a free state.
This law can be expressed the formula:
ΔМi =Zi . Mp + (Ai — Zi) . Mn — Mi (3.1)
Where ΔМi— defect of weight of i kernel,
Zi — a charge of i kernel (quantity of protons in a kernel);
Mp — weight of a proton; Mn — weight of a neutron;
Ai — mass number of i kernel (quantity of protons and neutrons in a kernel);
Mi — weight of i kernel.
The energy allocated at the formation of i kernel from protons and neutrons, is defined under Einstein’s formula:
Ei = ΔМi . C2 (3.2)
Where, C — speed of light.
At the synthesis of two kernels the formula (3.1)will look like:
ΔМ2с= Ma + Mb – Mc (3.3) Where, Ma, Mb — weights of two kernels a and b participating in the synthesis;
Mc (Mi) — weight of a kernel c received as a result of synthesis of kernels a and b.
ΔМ2с (ΔМi) — a part of weight allocated in the form of radiation. (Defect of weight at moment of synthesis of two kernels.)
The energy allocated in time of the synthesis of kernels a and b, is expressed by the formula:
E2с = ΔМ2с . C2 (3.4)
At the synthesis of three kernels the formula (3.1) looks like:
ΔМ3с= Ma + Mb + Md – Mc (3.5)
Where Ma, Mb, Md — weights of kernels participating in the synthesis;
ΔМ3с (ΔМi) — defect of weight in time of synthesis of three kernels.
The allocated energy at the synthesis of three kernels will be defined in the formula:
E3с = ΔМ3с . C2 (3.6)
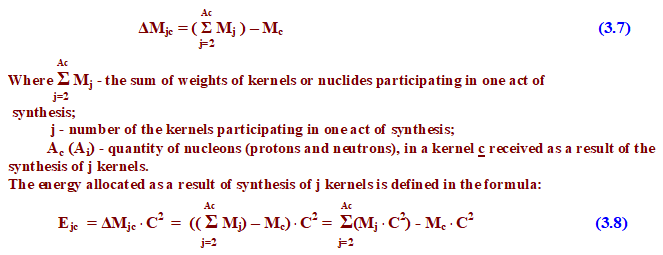
The general formula of defect of weight can be written down:
In the table № A-1 (in the application), the parameters of nuclear kernels are: M — the nuclear weight is given in Micro — U and in keV, Esv — the full energy of communication of kernels (keV) and
ΔD = (M-A) — a difference between nuclear weight and mass number of a kernel in keV. Surprising peculiarity of all these parameters is that for calculating the allocated energy Ejc during the time of synthesis of kernels in the formula (3.8) values (Mj . C2) and (Mc . C2) it is possible to replace with values ΔD or ((-1) . Esv), that simplifies the calculations. The formula (3.8) will look like:
For a variant of synthesis of two kernels this condition looks like:
Ac= Aa+Ab
Zc= Za+ Zb
Most conveniently and expediently is to use parameter ΔD, data in power units keV, that facilitates to us calculations.
Let’s check up the probability of replacing the parameters, on an example of synthesis of two kernels a and b in a kernel c.
Aa, Za, Ma — parameters of a kernel a, participating in synthesis;
Ab, Zb, Mb — parameters of a kernel b, participating in synthesis;
Ac, Zc, Mc — parameters of a kernel c, received as a result of synthesis of kernels a and b;
ΔDa, ΔDb, ΔDc — a difference between nuclear weight Mi and mass number Ai of kernels a, b and c (ΔDi = Mi — Ai).