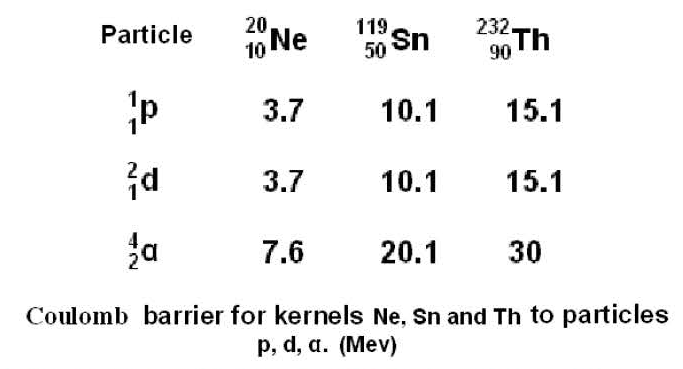
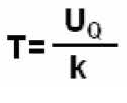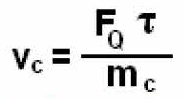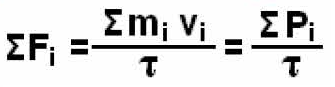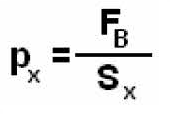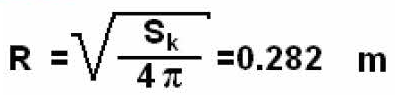(2)  Table №T-2.1                                  

For the synthesis of two kernels Sn it is necessary to overcome the height of coulomb barrier 261.3935 MeV; for two kernels No — 847.1 MeV
The sizes are huge, taking in account the tunnel effect these figures will be lower. But our purpose is to check up the possibility of synthesis of heavy and super heavy kernels in stars with not favorable conditions.
It is visible from the calculations that kernels with high kinetic energy can participate in synthesis.
Modern theorists believe that it is possible to receive kernels with such kinetic energy it is by warming them up to high temperatures.
As to thermal energy in 1 eV there corresponds temperature T ~ Calvin’s 110000 degrees

                                           U_Q= k ^. T                                                                     (2.11)         

The formula for calculating the temperature that is necessary for overcoming coulomb barrier:

                                                                                                 (2.12)

So for overcoming U_k ~ 0.1 MeV T~10^{9 o}K, it is assumed, that it in tens times higher than the temperatures inside of the Sun.

    

The values of these temperatures are simply fantastic. We shall not assort the variants with tunnel effect; the parameters will be high in this case as well. It is visible from the calculations, that inside of the stars, a synthesis of heavy kernels is impossible, as, temperature in stars is low. This is the conclusion that was made by physics theorists.
But practice shows that the synthesis of heavy kernels in stars exists. Hence, it is necessary to search for other solutions.
The variant of heating the substance of a star and definition of the necessary temperature for synthesis, by means of constant of Boltzmann does not inspire trust since it is developed for thermodynamic processes in gases, and thermonuclear synthesis is related to processes of nuclear where actions of thermodynamics laws are  limited by laws of nuclear physics.
Ifpractice and theory contradict each other, it is necessary to change the theory, instead of ignoring the practice.
There is a variant of synthesis at which the coulomb power barrier is absent. It is synthesis of a proton with a neutron, and kernels of atom with a neutron. In these cases the coulomb barrier doesn’t interfering the synthesis. Also there is an allocation of energy, without loosing it on resisting the coulomb forces. At synthesis of a kernel with a neutron the energy is allocated, then one of neutrons of a new kernel allocating electron, becomes a proton.

At such scheme of synthesis energy costs to overcome the Coulomb barrier is absent. Synthesis of one proton with neutrons is possible to Α = 3 at z = 1 and unstable isotope of hydrogen is possible to Α = 6. Consequently, the synthesis of hydrogen with Α = 6 and z = 1 is possible without the expenditure of energy, thus there is a release of energy for the synthesis of other nuclei.

                       2.2. Cold synthesis

Now scientists are increasingly talking about cold synthesis. In this case, fusion occurs at temperatures much lower than calculated from the formula(2.12)

                                                                                         (2.12)

For cold synthesis requires two conditions:
    1. Compress the matter to large densities, bringing the nucleus as close as possible to each other, preferably at a distance of nuclear forces.
    2. Report nuclei of the atoms speed necessary to overcome the Coulomb barrier:

                                                     P_c = m_c ^. v_c

 Where P_c — an impulse of a kernel,
             m_c — weight of a kernel,
             v_c — the speed of a kernel which is necessary for overcoming coulomb barrier.
                                             m_c ^. v_c = F_Q ^. τ
           F_Q — coulomb force,
           τ <1 sec. — time. 

                                                        

Is «cold synthesis» is possible in stars?
Let’s consider the experience of human under the decision of a problem of synthesis of kernels.
The creation of huge temperatures, in billions and hundred billions degrees while is impossible. But, the human synthesizes kernels of atoms of periodic system, without heating to such temperatures.
We will consider ways of synthesis of kernels of atoms applied by the human.
   The first way: Accelerators of particles.
Particles, nucleons, protons or easy kernels are dispersed by means of electromagnetic fields and aimed to plates where new kernels of atoms are formed after collision. That means, the particle is dispersed till the speed which will allow to overcome the coulomb barrier and inserted into a kernel.
   The second way: It is used in a «hydrogen» bomb. A vessel with tritium and deuterium is being surrounded with a uranium nuclear bomb and blown up.
As a result of the explosion of a uranium bomb, the part of the blown up substance takes off outside, and other part, under the third law of Newton, with the same force compresses deuterium and tritium, that gives huge speed to kernels. But, it already influenced volumetric, and directed to the central area that strengthens the action of compression.
As a result we receive synthesis of kernels of atoms at temperatures that are lower than those calculated in the formula (2.12).
A natural question arises, «If human could bypass a theoretical condition, why the nature can’t?»
Why the human considers, that the nature is sillier than him?
Further we shall see that the nature not only is not sillier than the human, many times it is far more rational and economical. Analyzing the schemes and processes operating in space,we shallunderstand, on how many all is created ingeniously, and that proves unequivocally the existence of the Supreme reason — reason of Our Creator.
The mankind approaches to the level of knowledge, «knowledge Supreme, through the knowledge of its creations».
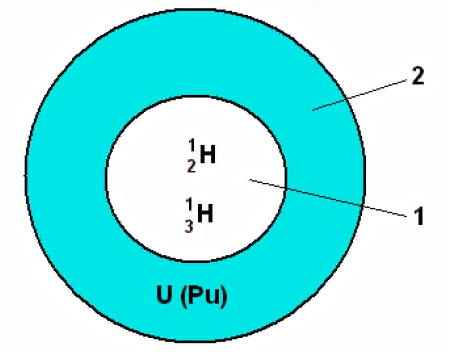
Let’s disassemble the scheme of a hydrogen bomb, in more detail.

             

1 — area of an arrangement of easy kernels, deuterium and tritium,
2 — area of an arrangement of a uranium bomb – «detonator».
«The scheme of a hydrogen bomb»
(3) Figure № R-2.1.

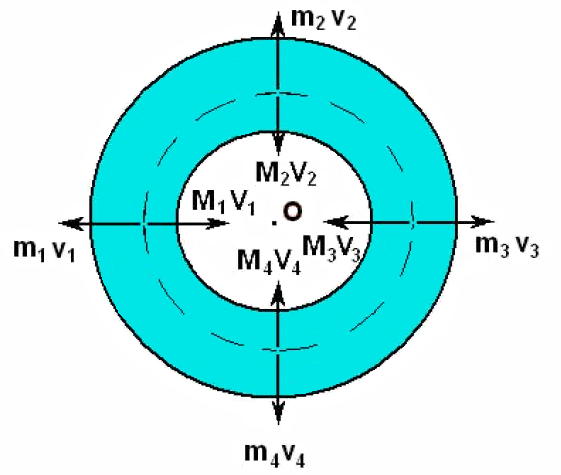
At explosion of a uranium bomb, a part of the explosive with weightsm₁, m₂, m₃, m₄, scatter with speeds v₁, v₂, v₃ and v₄ in the different and opposite parties from the center with impulses P₁ = (m₁ ^. v₁); P₂ = (m₂ ^. v₂);        P₃ = (m₃ ^. v₃) and P₄ = (m₄ ^. v₄) as shown in figure № R-2.2.

The action principle of a uranium detonator on a volume with deuterium and
tritium.
(4) Figure № R-2.2.

Other part of an uranium detonator with weights M₁, M₂, M₃, M₄ according to the third law of Newton with same impulses Р₁, Р₂, Р₃, Р₄ will move to the center of a bomb to a point «O» with speeds V₁, V₂, V₃, V₄. That means, according to third to the law of Newton, the following expressions will be fair:
                        P₁=m₁ ^. v₁=M₁ ^. V₁;     P₂=m₂ ^. v₂=M₂ ^. V₂;
                        P₃=m₃ ^. v₃=M₃ ^. V₃;     P₄=m₄ ^. v₄=M₄ ^. V₄
Hence, according to the forces with whom the splinters of an uranium detonator will scatter, it is possible to define force of compression of deuterium and tritium:
                                             P_i=m_i ^. v_i=F_i ^. τ
  Where τ — time,
         F_i — force of compression from impulse P_i, received m_i.

                                                                             (2.13)

General force of compression of area 1 (fig. № R-2.1) will be equal

                                                                (2.14)

Not an unimportant role in the process we check is the form of the bomb — the «Sphere». This form gives concentration of force in the central area, which strengthens the compression of a hydrogen component of the bomb, and, hence, synthesis of easy kernels.

(5) Figure № R-2.3
«The spherical form of a hydrogen bomb»

                      R₁ — radius of a sphere with deuterium and tritium,
                      R₂ — radius of all bomb,
                      H_U = R₂ — R₁ — thickness of an environment from uranium or wandering,
                      R_a — radius on which force ΣF operates.
Since we’re interested in the force which operates inside sphere, we shall designate it F_B = ΣF
From the formula 

                                                     F = p ^. S

  Where p — pressure, and S — the area of action of force F.
It is possible to define pressure of compression of area 1 (fig. № R-2.1) on distance Ra action of force F_B

                                                   F_B= p_a ^. S_a                                                                               (2.15)

  Where S_a = 4 ^. π ^. R_a² — the area of sphere with radius R_a

              p_a — pressure of compression, on distance R_a from the center of the sphere.
As approaching the center of the sphere to point «O», the radius of a sphere decreases from value R_a and aspires to zero. Hence, the area of the sphere, will decrease with the approach the center, from value S_a to zero. But since the force of compression enclosed on distance R_a, does not vary, hence, with the decrease in the area of the sphere surface in the formula (2.15) pressure p will grow.

                                                                                           (2.16)

  Where S_x — the area of sphere with radius

         R_x = O ~ R_a

At radius R_k the area of a surface will be S_k= 1 m² pressure P_x = P_k
Will accept the value of force F_B

                                                                        (2.17)

That means, at radius 

                                  

The force which compresses all area 1 (fig. № R-2.1) will act on the area in 1 m².
At radius less than R_k, the pressure will increase, aspiring to infinity, but being limited to the sizes of a nuclear kernel in the center of the sphere in point «O».